The Geometry of Bourges Cathedral: A Step-by-Step Reconstruction.

One logical strategy for the first designer would have been to start with the available space for his new chevet. He could have begun, therefore, by striking a semicircle like the one shown here in red, with a radius of 24.35m; in the finished building, such a circle is tangent both to the main buttresses faces of the chevet and to the exterior faces of the small radiating chapels.

Next, after deciding that the chevet should have five equal wedges, the designer could have divided the red semicircle into ten smaller wedges of 18 degrees each (only four of which are shown here, for the sake of simplicity). The orange perpendicular from the first wedge intersection point to the baseline of the hemicycle will stand 23.16m from the geometrical center, where in modern parlance we would say that 24.35m x cosine(18°) = 23.16m. The length of this orange perpendicular is 24.35m x sine(18°) = 7.53m, which becomes the radius of the hemicycle. Equivalently, we can imagine that the designer may have begun with the radius of the hemicycle, which the designer of Notre-Dame in Paris seems to have done, before going on to use the 18-degree symmetry of the wedge composition to establish the red outer circle. The relationship between the 24.35m and 7.53m dimensions remains the same in either case.

As the yellow lines now show, the interval between the orange perpendicular and the outer red circle equals the radius of the pier plinths. In numerical terms, this interval is 24.35m – 23.16m = 1.19m. This interval will go on to play many important roles in establishing both the plan and elevation at Bourges. It is interesting and significant that the Bourges designer paid attention to plinth widths as well as pier axes, since similar relationships between plinth width and the overall chevet geometry can also be seen at Notre-Dame in Paris, and since plinth-to-plinth measurements figure prominently in the Florentine buildings studied by Matthew Cohen (Cohen 2008; Cohen 2014a; Cohen 2014b).

As the green lines here show, a perpendicular dropped from the second intersection point on the large red circle will intersect the chevet baseline at a distance of 19.70m from the center, where 24.35m x cosine(36°) = 19.70m; this will be the distance from the hemicycle center to the plinths of the shaft bundles embedded in the outer wall. Meanwhile, a perpendicular dropped from the first wedge intersection with the hemicycle will stand 7.53m x cosine(18°) = 7.16m from the hemicycle center. The interval between these most recently constructed perpendiculars is 19.70m – 7.16m = 12.54m, and the midpoint of the interval will be 13.43m from the building centerline. It would have been easy for the designer to use this interval as the baseline of the two aisle bays, and the 13.43m dimension as the span to the intermediate pier centers. Indeed, the length of the interval precisely equals the sum of the two notional aisle widths identified by Tallon: 12.54m = 2 x 6.27m. Instead of using the interval in the place where it arises naturally, though, the designer displaced it slightly outward. The reasons for this displacement will have to be considered more carefully near the end of this analysis, but the magnitude of the displacement can be understood at once.

As the blue lines on the right of the image indicate, the interval seen on the left has simply been displaced, so that its inboard endpoint lies on the circle of the hemicycle. The center of the interval thus falls 7.53m + 6.27m = 13.80m from the building centerline, aligned with the centers of the intermediate columns, and the outboard point of the interval falls 7.53 + 12.54m = 20.07m from the centerline, aligned with the inner surface of the outer wall. The steps discussed so far thus suffice to generate the three circles measured by Tallon (Tallon 2014).

With these results in hand, the outer wall surface can easily be found by adding one plinth radius of 1.19m to the inner wall surface; the outer surface thus lies at a distance of 20.07m + 1.19m = 21.26m from the hemicycle center. More details could be added to this picture, but the outlines of the plan shown here give enough information about the pier and wall placement to permit analysis of the elevation scheme that grew from it.

Here we see the scan of the eastern straight bay, following Tallon’s laser-scan data.

The geometrical baseline of the elevation corresponds to the original medieval floor level, which lies 30cm below the present floor (Tallon 2014). This graphic also shows the main axes already established in the ground plan, whose distances from the centerline are as follows:

• 7.53m for the arcade axis
• 13.80m for the intermediate pier axis
• 20.07m for the inner face of the outer wall
• 24.35m for the outer face of the buttress

Next, in orange, come the secondary axes, whose distances from the centerline are as follows:

• 19.70m for the inner face of the engaged shaft bundles
• 21.26m for the outer face of the outer wall

Recalling from the plan that the plinth edges stand 1.19m from the axes, we can add lines:

• 6.33m to the inside of the arcade plinth
• 8.72m to the outside of the arcade plinth
• 12.61m to the inside of the intermediate plinth
• 14.99m to the outside of the intermediate plinth

To these we can readily add the following:

• 10.66m = (8.72m + 12.61m)/2 for the middle axis of the inner aisle
• 17.34m = (14.99m + 19.70m)/2 for the middle axis of the outer aisle
• 20.66m = (20.07m+ 21.26m)/2 to the midline and glass plane of the wall

With those preambles out of the way, we can begin to consider the elevation, as such, starting with consideration of ‘ad triangulum’ geometries. As Andrew Tallon has noted, equilateral triangles framed by the outer and inner wall surfaces cannot easily explain the cathedral’s vault height, since they are too tall or too short, respectively (Tallon, 2014).

A yellow equilateral triangle framed by the midlines of the walls, though, rises to a height of 35.79m, beautifully matching the interior height of the main vessel up to its keystone.

It makes sense that the vault height should relate to the span between the midlines of the walls, because these midlines locate the glass panes in the outer aisle windows. The windows delimit the interior space of the cathedral in the horizontal dimension, just as the vaults delimit it in the vertical dimension. The way the yellow triangle relates these components offers powerful evidence for the use of ‘ad triangulum’ planning at Bourges. But the yellow triangle was by no means the only one that the first Bourges designer used to establish the elevation of his cathedral. As the lower left section of this graphic shows, for example, the intersection of the inner green triangle with the outer pier axis sets the 10.86m height of a horizontal drip molding on the pier buttress.

More strikingly, perhaps, a large red equilateral triangle framed by the outer edges of the buttresses reaches a height of 42.18m, establishing the height of the pinnacles on the gutteral wall.

Further evidence for the unity of the choir design comes from consideration of the timber roof, whose peak fits neatly into a great square framed by the outer buttress edges. The whole section of the choir, therefore, elegantly relates the ‘ad quadratum’ and ‘ad triangulum’ geometrical schemes. So, while the upper choir walls may well have been constructed by a follower of the original master, as Robert Branner suggested (Branner 1989), the coherence of the whole choir section provides strong evidence for the unitary conception of its geometrical framework.

The details of the section also develop neatly within this frame. To see how this works, it will prove useful to consider not only the red, orange, yellow, and green triangles discussed previously, but also the blue triangle seen here, whose base is framed by the plinths of the engaged shafts on the outer wall, and whose apex lies at a height of 34.12m, well below the keystones of the main vaults.

The top of the inner arcade lies at height 21.09m, where this blue triangle crosses the vertical axis of the inner aisle pier. As the lines of 30-degree slope from the corners of the large red triangle indicate, this level also marks the midpoint of this red triangle that locates the pinnacles at height 42.18m. These two heights match only because of a subtle relationship established in the ground plan: as the previous analysis of the plan showed, the widths of the vessels and the placement of the vertical axes all depends on the decagonal symmetry of the chevet. This matters because decagons and pentagons incorporate remarkable dimensional relationships based on the Golden Section. In this particular case, the crucial point is that the half-width of the choir vessel, 7.53m, relates to the radius of the whole chevet by a factor of sine (18°) = .309, while the 17.90m half-width of the blue triangle relates to the radius of the chevet by a factor of cosine (36°) = .809. The surprising fact that the difference between these is exactly .500 means that the portion of the blue triangle between its lower corner and the arcade axis will be exactly half as large as the side of the large red triangle. Although the designer of the Bourges choir would not have used the modern language of trigonometry to describe this relationship, he clearly understood enough about compass-based geometrical constructions to establish it in his building, thus knitting the logic of the plan and elevation together in a highly sophisticated manner.

Several of the other levels in the choir section can be found quite readily within this frame. The sloping side of the large red triangle cuts the glass plane of the outer wall at height 6.39m, for example, thus establishing a level marked by another drip molding on the outer buttress. The height of the outer aisle columns, measured to the tops of their capitals, is 4.71m, which is equal to the width of the aisle measured between its plinths. The top of the aisle story is twice as high, at level 9.42m, as the blue diagonals in the lower left of the graphic show. A nearly equivalent ‘ad triangulum’ scheme can be seen at right; the green triangle crosses the middle axis of the outer aisle at height 4.72m, differing from the version at left by a practically negligible 6mm. The analogous ‘ad triangulum’ system certainly seems to have been used in the inner aisle, where the top of the capitals falls at height 16.30m.

A closely related multi-step process can be used to establish the tops of the main vault capitals, at height 26.52m. The first step in the process is to note the intersection between the blue triangle and the inner aisle axis at height 15.66m. The interval between this height and the top of the arcade, which is 21.09m – 15.66m = 5.43m, can then be flipped up above the arcade, as the circle in the middle of the figure indicates, to reach the new height of 21.09m + 5.43m = 26.52m.

The upper chords of the flying buttresses converge at the apex of the large red triangle, at height 42.18m. This level, which coincides with the height of the pinnacles, seems to have been understood as the upper margin of the cathedral’s masonry structure, while the timber roof rises higher to meet the great square that shares its baseline with the red triangle. The slope of the flyer chords can be found by connecting the triangle’s apex to the points on the outer buttress margins at height 16.22m, a level established by the intersection of the triangle’s side with the vertical rising from the column plinth 14.99m from the building centerline.

The top of the buttress upright lies at height 20.69m, the level where the sides of the large yellow triangle cut the vertical rising from the outer plinth of the main arcade pier (more precisely, this level marks the top of the original buttress uprights, before the pinnacles and extensions were added in the 19th century). To find the intrados of the lower outer flyer, begin by establishing the height 11.78m, half an aisle span above the outer aisle. Next, establish the vertical defining the inner face of the buttress upright, which is halfway between the window plane and outer wall surface, located 20.66m and 21.26m from the building centerline, respectively. The orange semicircle whose outer portion describes the buttress intrados has a diameter at height 11.78m that spans between this new vertical and the axis of the inner arcade pier.

The construction of the upper outer flyer proceeds similarly, as the yellow lines in the graphic show. This time, however, the diameter spans all the way from the buttress margin to the building centerline, and it sits at height 14.50m; this is the level where a diagonal starting on the inner arcade axis at the previously established height 9.42m reaches the vertical rising from the plinth of the outer column. The same 14.50m height marks the top of the aisle triforium.

The center point of the lower inner flying buttress can be found at height 22.24m, halfway between the points where the large yellow and the green triangles intersect the axis of the inner arcade pier. The center point of the flyer lies directly below the point at height 26.52m where the large orange triangle crosses the horizontal at the top of the capitals. The radius of the flyer extends out to the inner face of the buttress upright rising from the aisle wall, which is 7/8 as wide as the plinth of the pier below, as the red diagonal construction in this zone indicates. Yellow lines, meanwhile, show that the top of the aisle wall lies at height 22.44m, halfway between the base of the upper triforium and the intersection between the large blue triangle and the orange vertical centerline of the flying buttress arch.

The arcs describing the intrados of the inner upper flyers are actually segments of a large circle, shown here in yellow, whose center lies on the building centerline at height 19.49m, the level where the large yellow triangle cuts the 30-degree line sloping up from the corner of the large red triangle to meet its midpoint.

The top chords of the lower inner flyers are less steeply sloped than the upper ones, so that the lines describing both sets converge at the same points on the outer surfaces of the buttress uprights, at height 16.22m. The lines describing the lower flyers converge on the building centerline at a height of just over 37m. This graphic shows one hypothetical way to reach that level, with small green circles centered at height 36.82m, aligned with the top of the large orange octagon. Each circle has a diameter of 1.19m, equal to the radius of one of the pier plinths at ground level. Blue verticals rising from the centers of the circles describe the centerlines of the pinnacles. The top points of the circles lie at height 37.42m, closely aligned with the bottom horizontal beam in the timber roof; the sloping sides of the roof also converge to points at this height on the main pier axes. When small squares are inscribed within the circles, their top edges lie at height 37.24m, which has been chosen here as the convergence point of the blue lines describing the lower flyer chords (it is difficult to be more precise about this, because the north and south buttresses differ very slightly in their slopes and articulation).

The upper chord of the lower outer flying buttress coincides with a line that begins to rise from the outer margin of the buttress upright at height 12.93m, coinciding with the level where the large orange triangle cuts the rising vertical axis of the outer column. The chord aims toward a point on this vertical axis at height 21.73m, aligned with the level where the green triangle cuts the inner column axis.

The tops of the column bases fall 0.84m above the height of the original floor; this height is smaller than the 1.19m column plinth radius by a factor of √2, as shown by the construction of the green octagons within the diagonals descending from the plinths. Meanwhile, red verticals rising just beyond the exterior wall surfaces of the lower aisles show moldings along the buttresses that stand roughly 0.30m out from the wall surface; this interval should probably be understood as one quarter of the 1.19m plinth radius. This graphic, in sum, makes visible a single coherent geometrical framework that seems to have governed the design of the Bourges choir from its overall outlines down to the size of its details. The nested equilateral triangles of the ‘ad triangulum’ scheme were, of course, among the most important elements in this framework, but the Bourges designer also took care to make sure that his building would fit neatly within a framing square when its roof was included, and he developed its plan from the five-fold subdivision of the chevet. His design, in other words, elegantly knitted together geometries based on the numbers 3, 4, and 5. There is good reason that he may have been thinking in terms of the number 8, as well. After all, the piers at Bourges each have eight shafts, instead of the more typical four. But the more powerful argument for the relevance of 8 is geometrical.

A great octagon whose lower facet coincides with the original floor of the central choir vessel reaches a height of 36.33m. This level, near the top edge of the vaults, corresponds to the level where the wall steps outward just above the clerestory windows. The corner of the octagon, at height 10.64ms, also coincides with the bottom of the molding on the exterior wall of the outer aisle, one of the few details not accounted for in the previous graphics. If the Bourges designer did indeed mean for the proportions of his main vessel to approximate those given by the great octagon, this might help to explain why he took a curious choice in the establishment of the cathedral’s ground plan.

As noted in the discussion of the plan, the 19.70m distance arises naturally from the subdivision of the chevet into five equal wedges. It would have been easy for the designer to use this distance as the span from the chevet center to the inner surface of the outer wall. Instead, though, he placed the inner wall 20.07m from the center, a distance that arises only through a somewhat awkward process of geometrical displacement: the 12.54m interval that arose as the difference between 19.70m and 7.16m, as seen on the bottom left of this figure, has to be pushed outward so that it fills the space between 7.53m and 20.07m from the centerline, as seen on the bottom right. It is at least conceivable that the designer’s decision to move the interior wall outward to its present location may have been motivated by a desire to expand the geometrical baseline of the ‘ad triangulum’ scheme, so that its height would more closely approximate that of the octagon scheme. In this hypothetical scenario, the designer’s consideration of the elevation would be feeding back into the design of the ground plan. Such a process would stand in contrast to the usual picture of Gothic design practice, in which information flows only from the ground plan into the elevation. It is plausible, however, because many Gothic designers surely had at least some rough ideas about their buildings’ elevations even before they set out to draw their ground plans, and because the first Bourges designer seems to have been particularly interested in synthesizing multiple design schemes into one tightly interwoven composition.

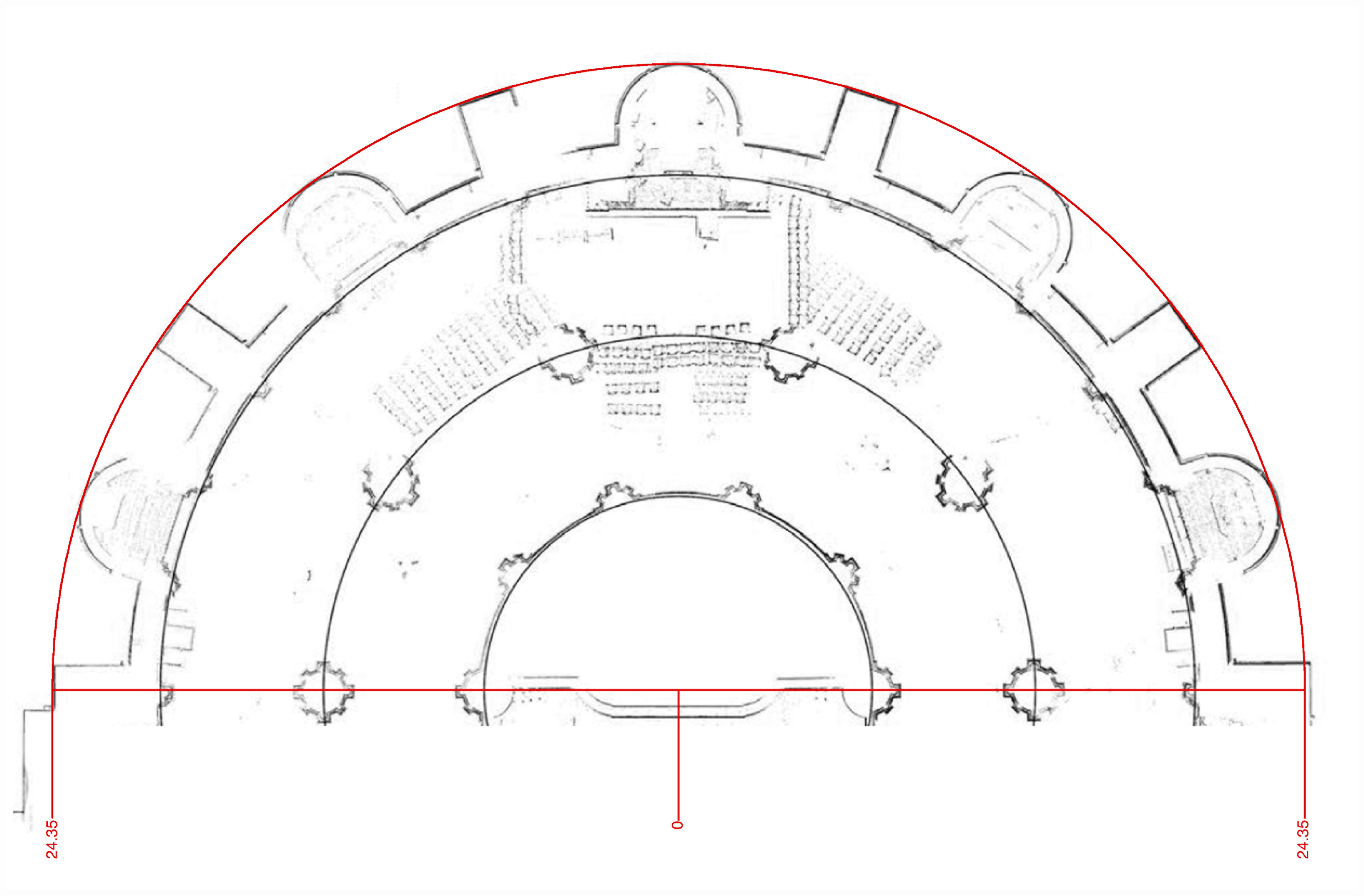
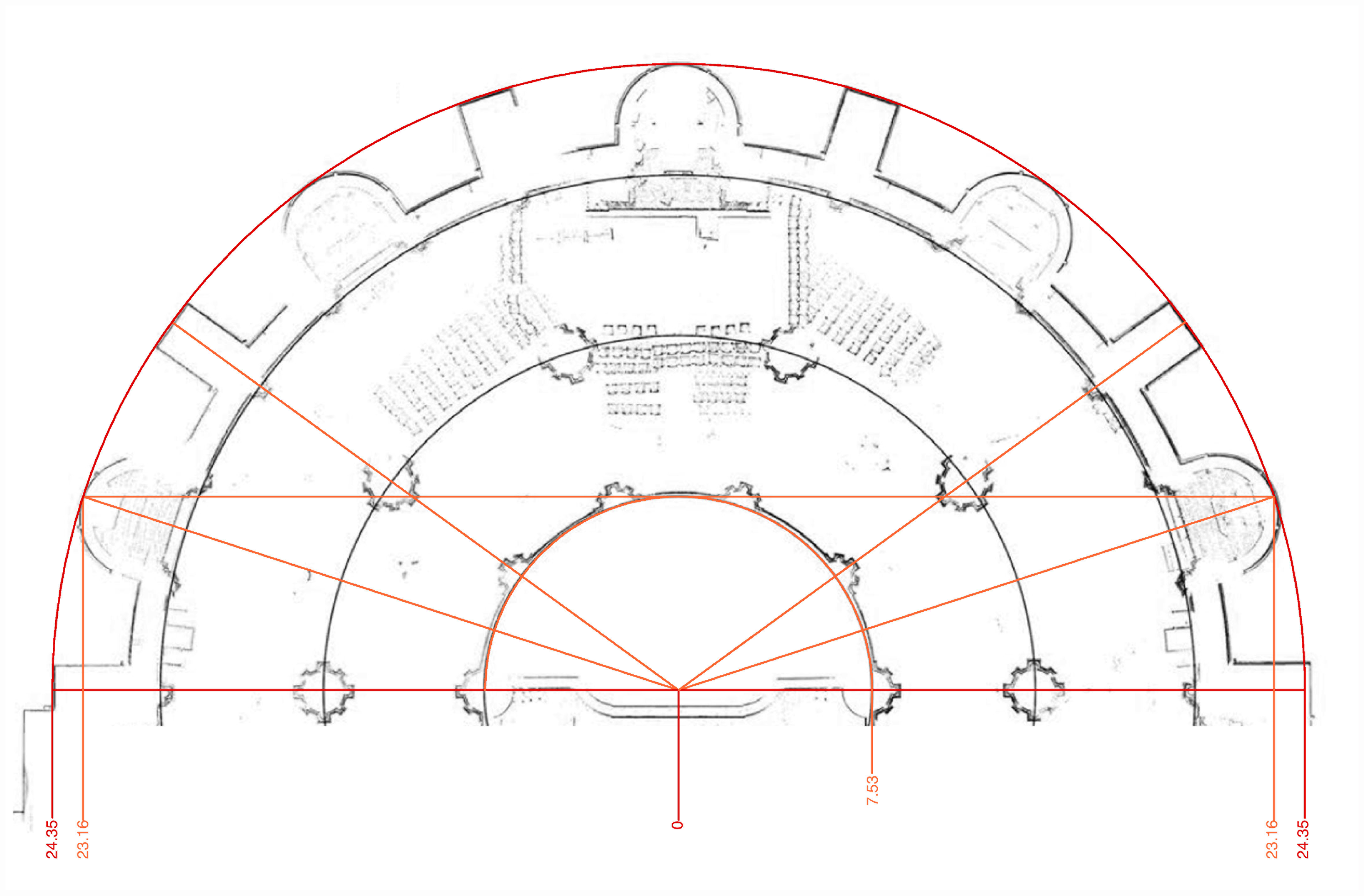
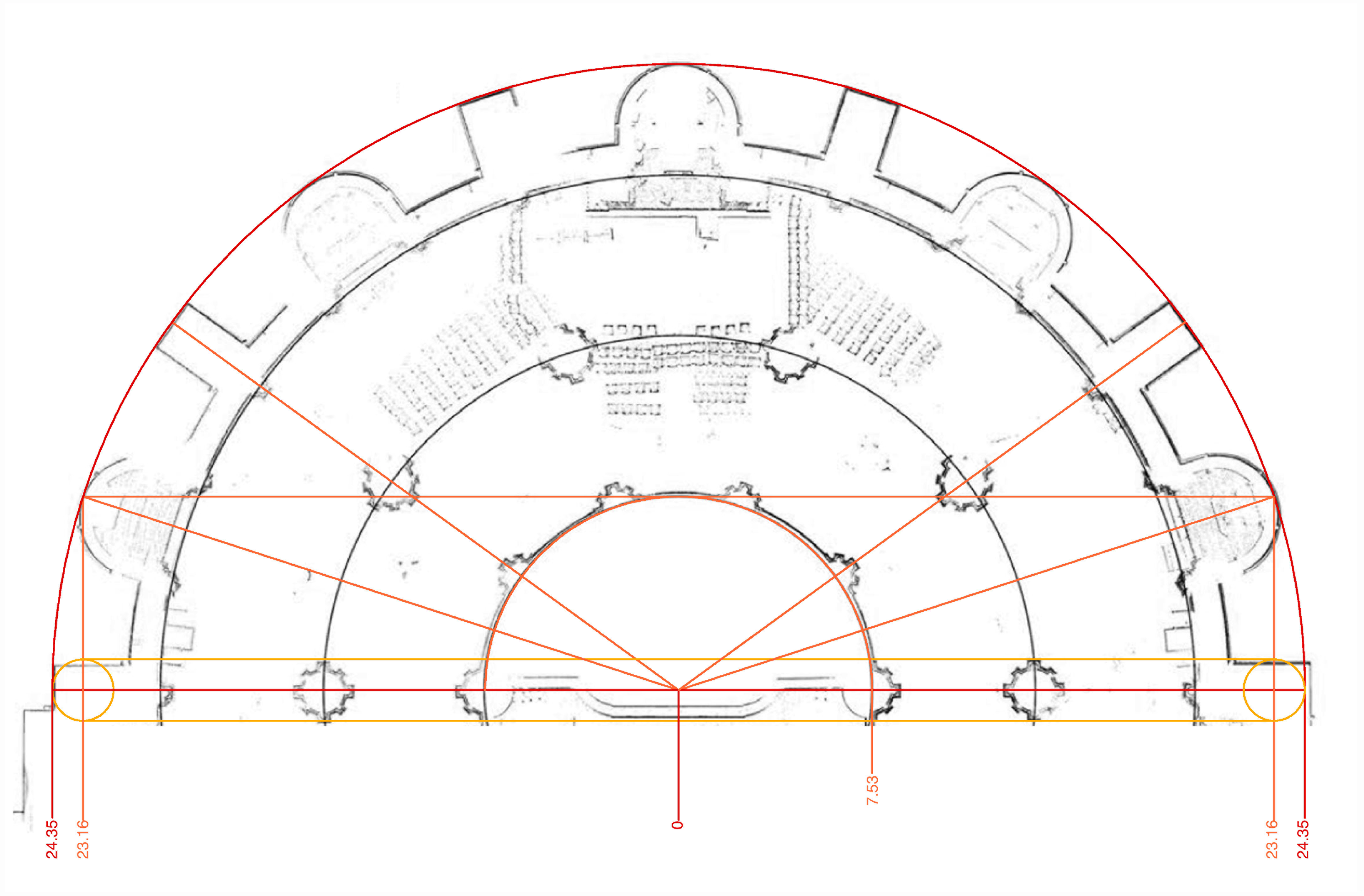
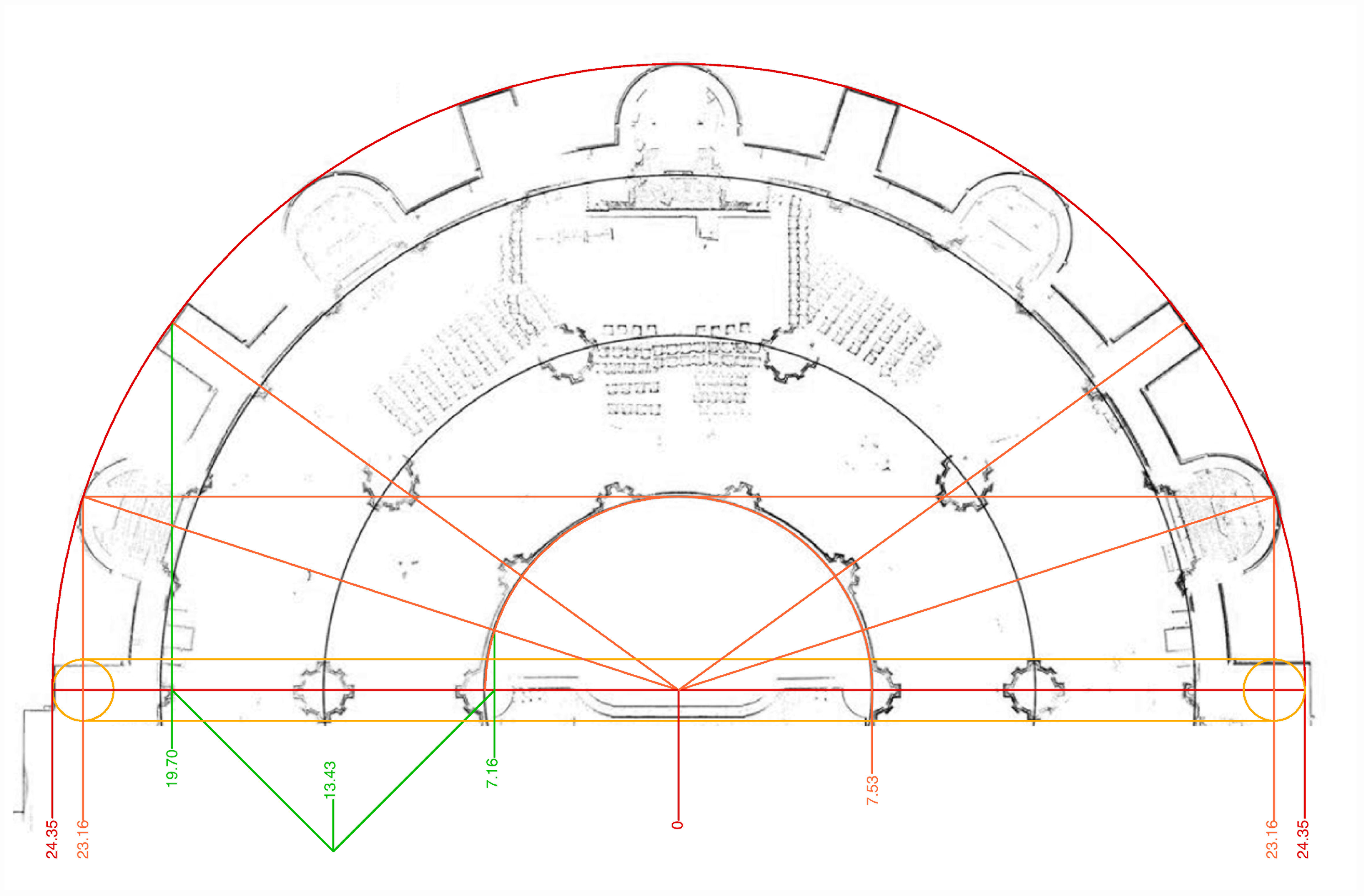
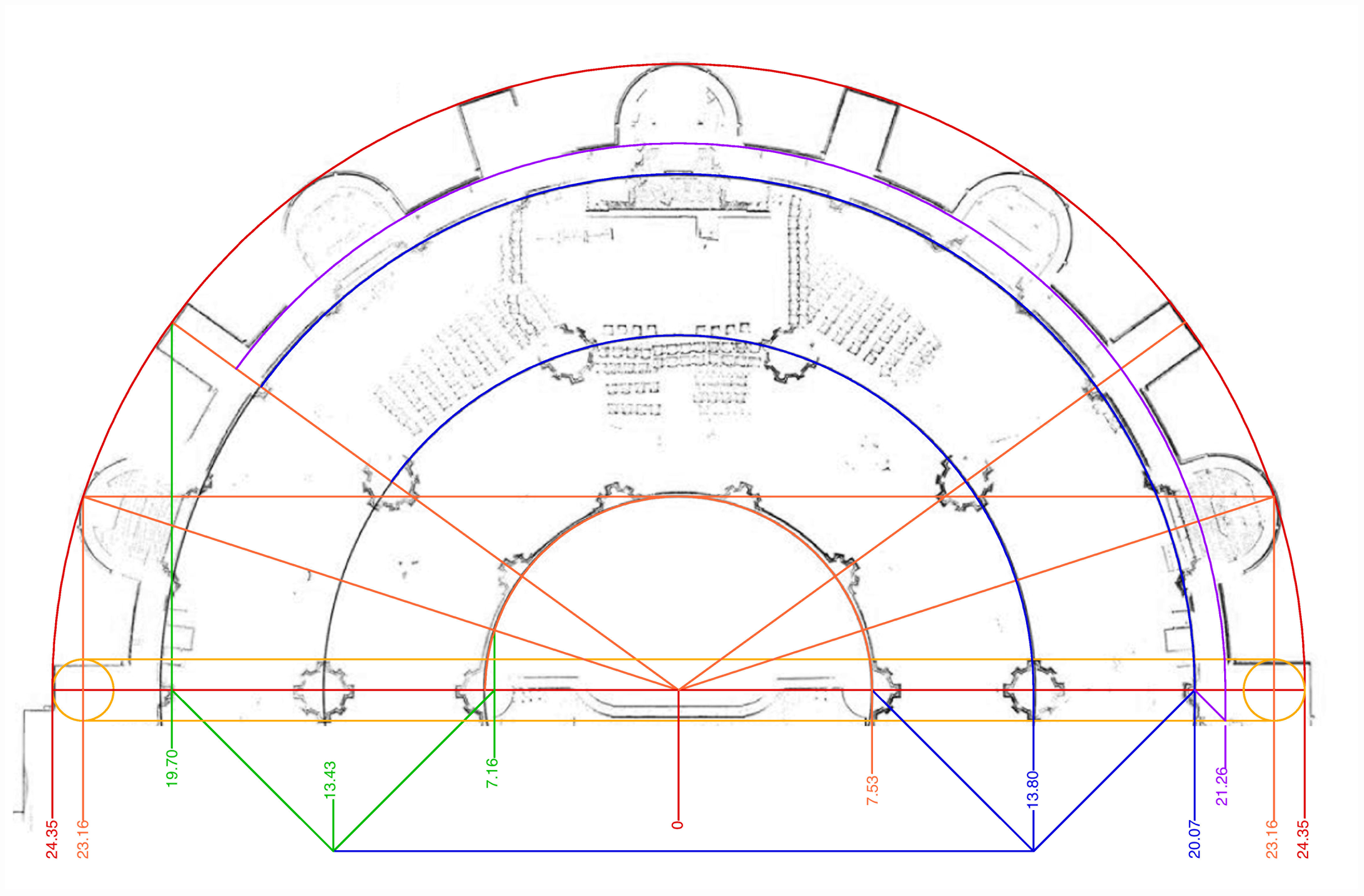
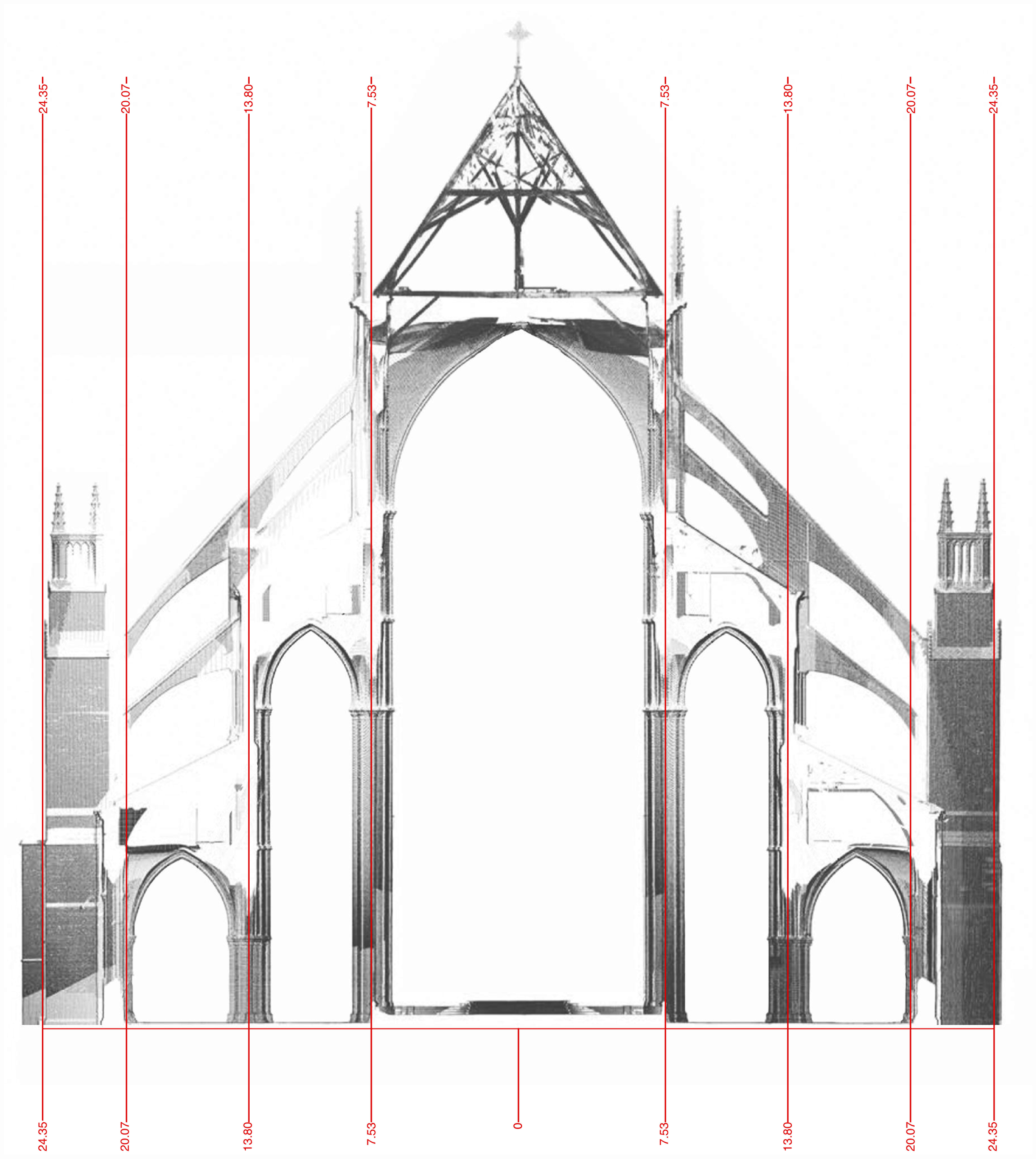
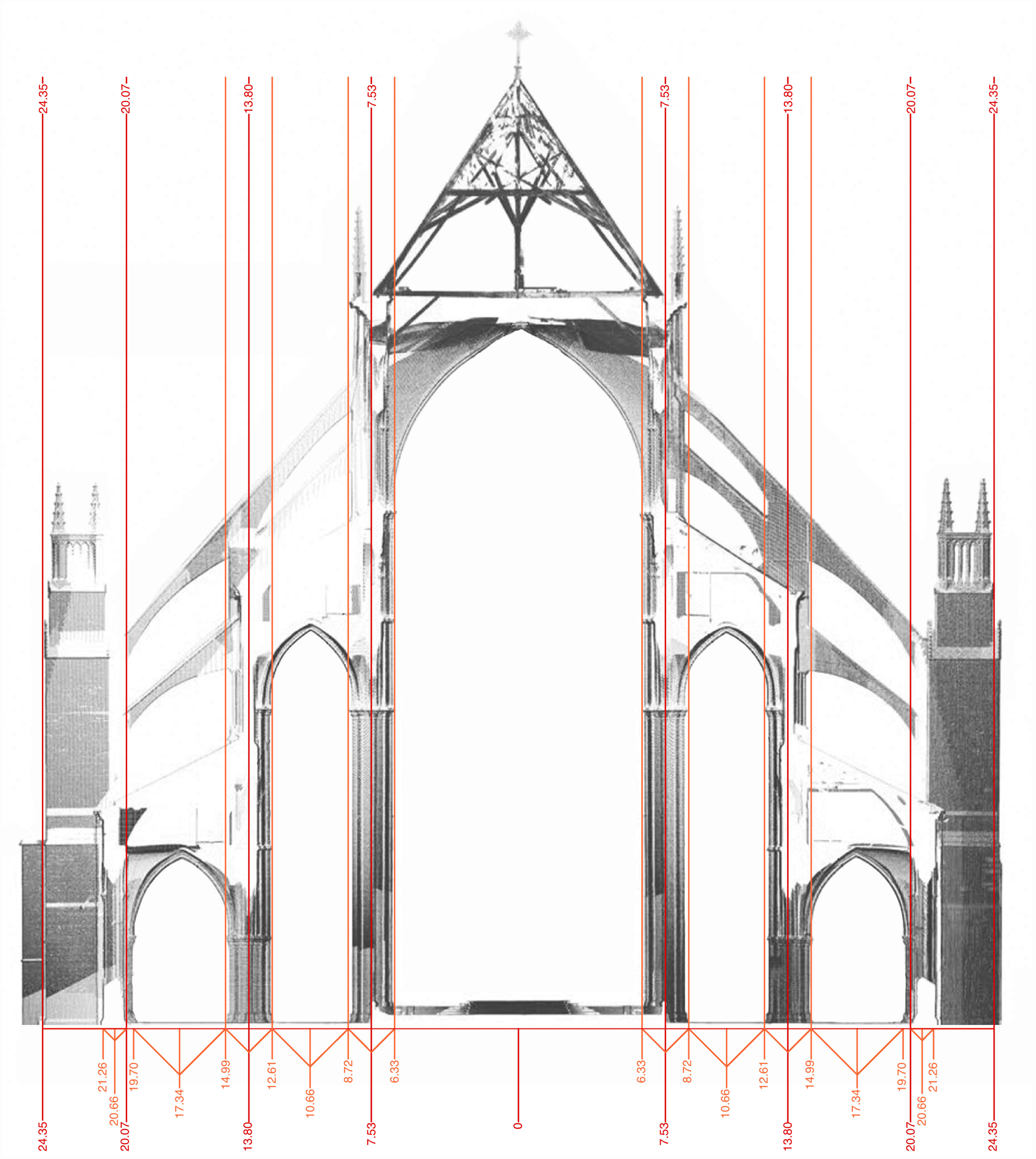
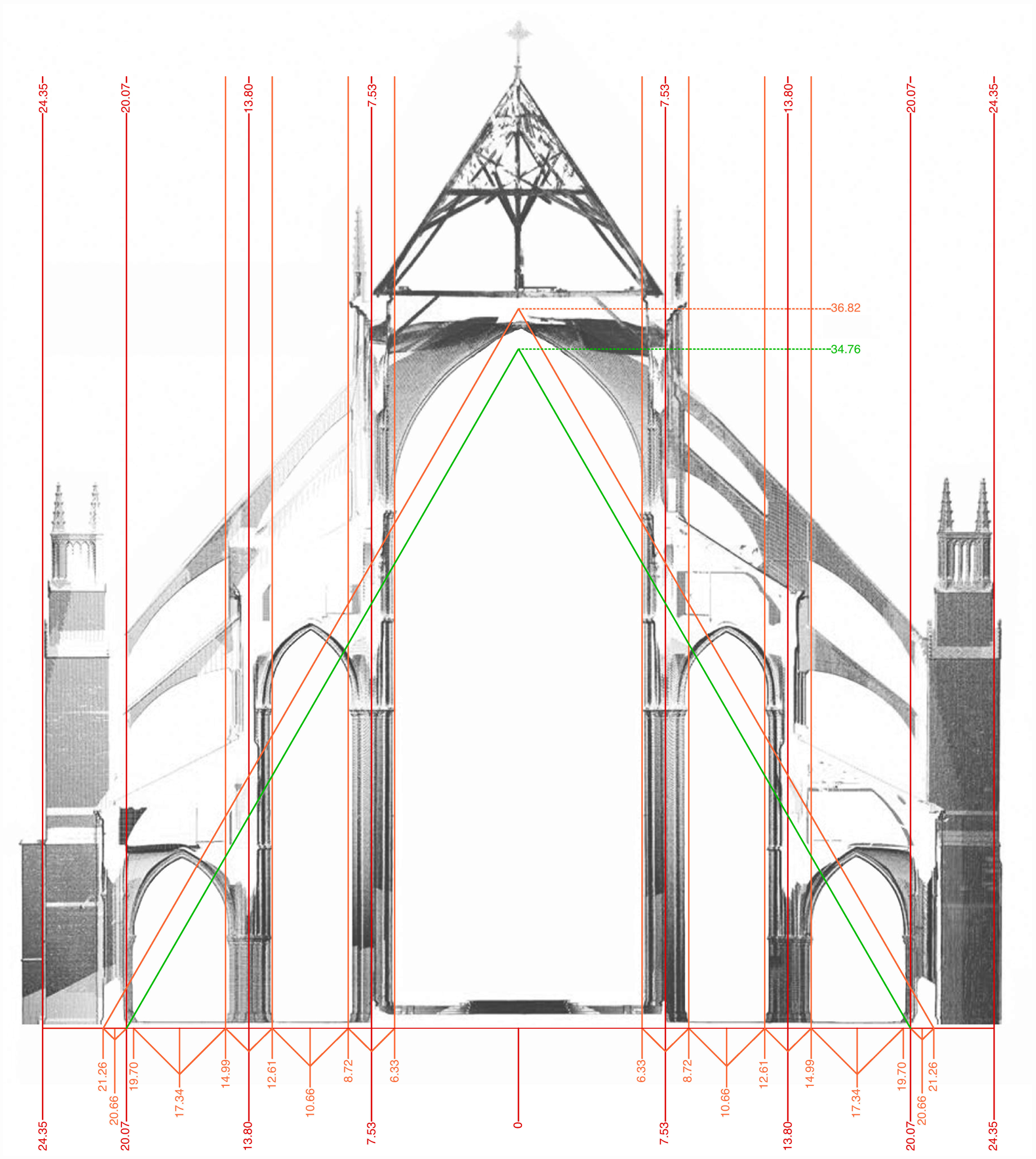
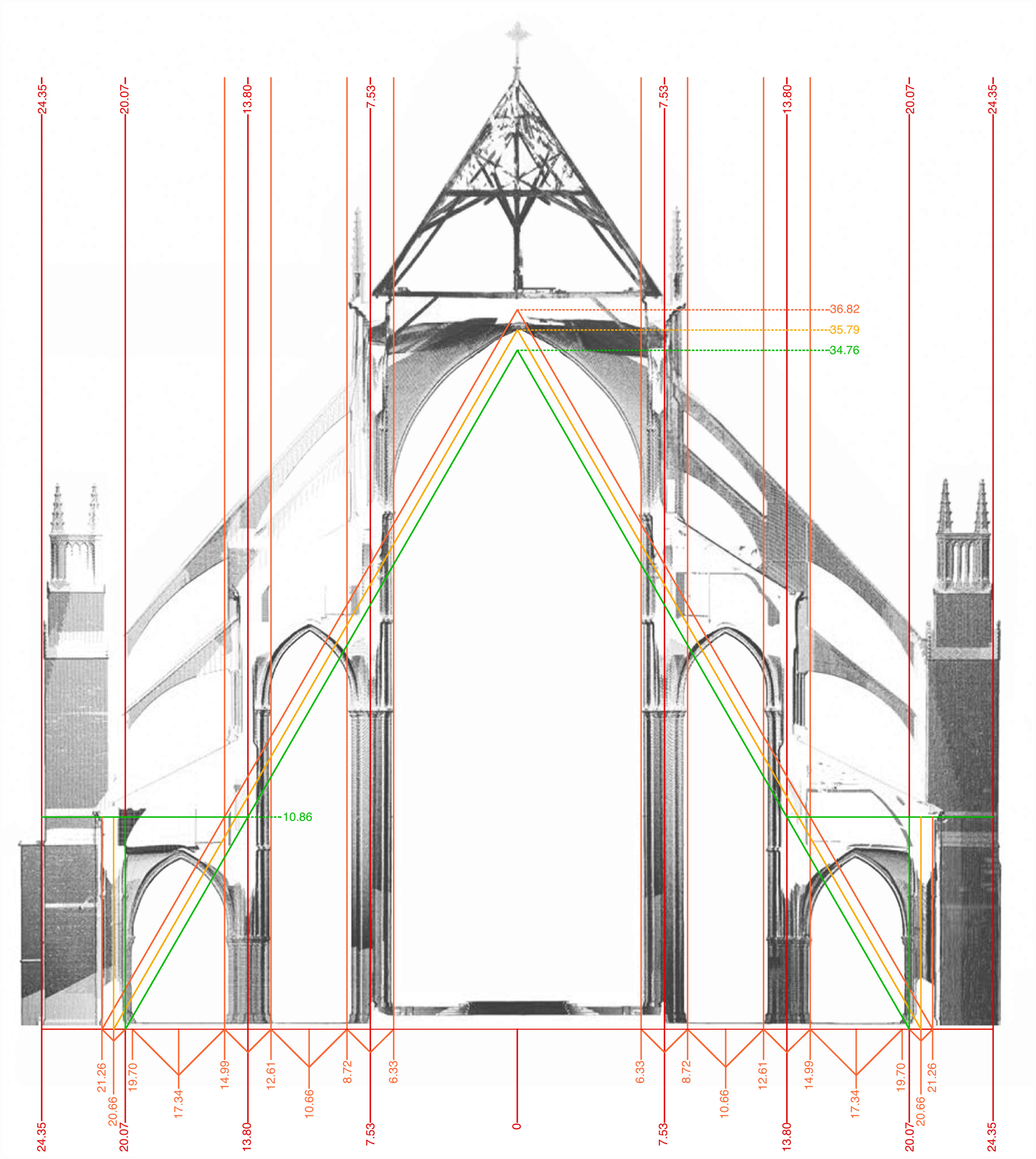
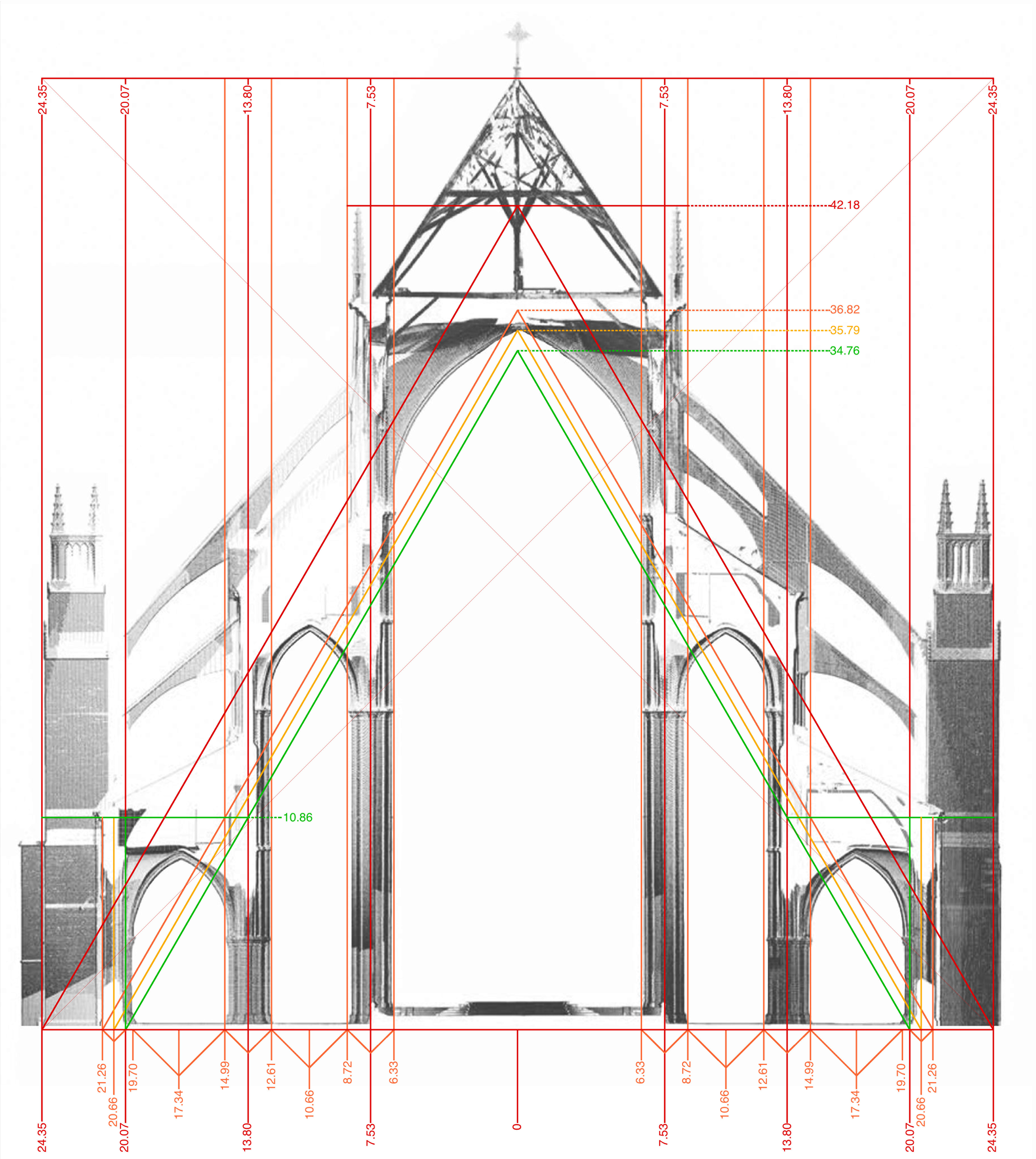
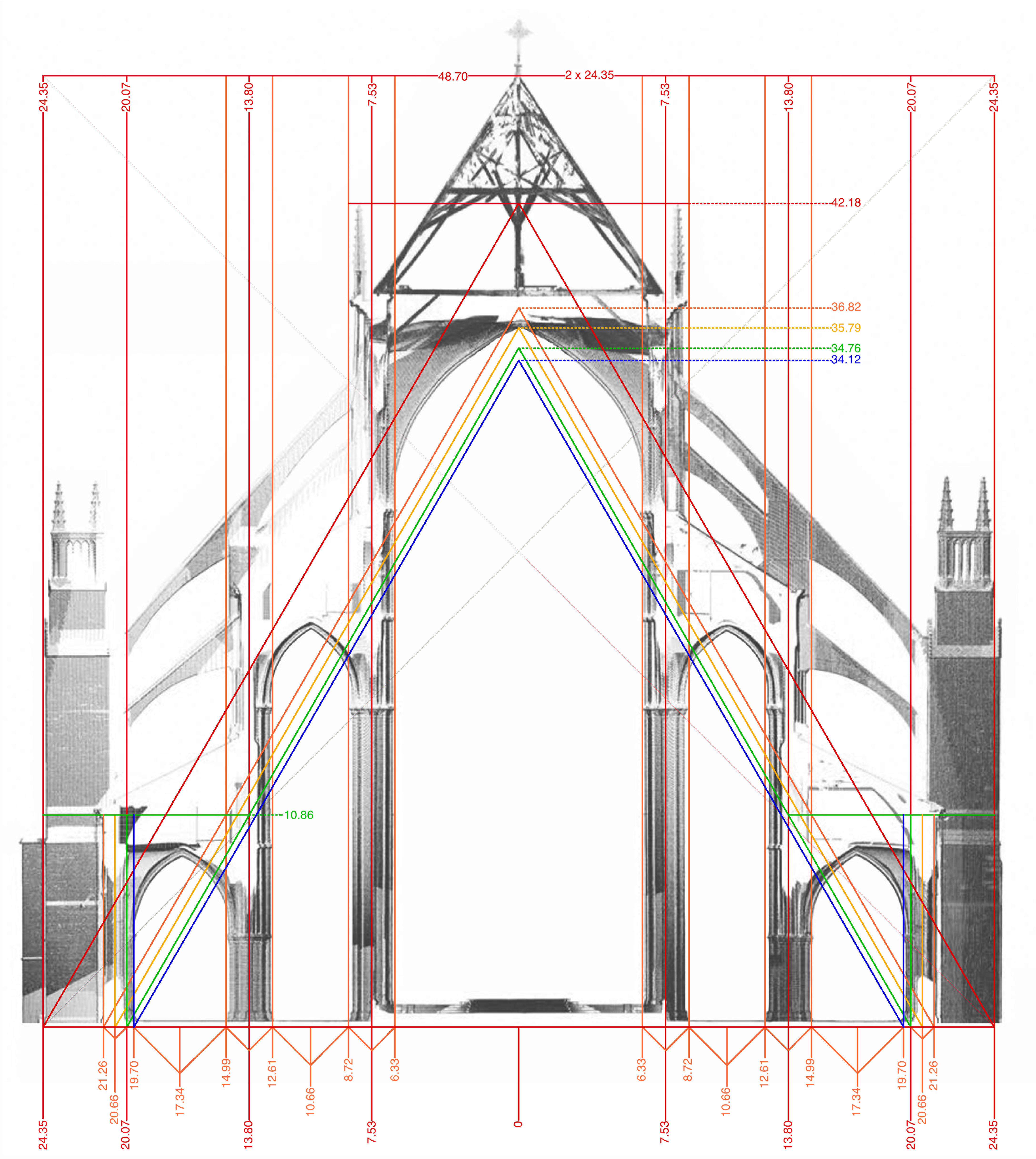
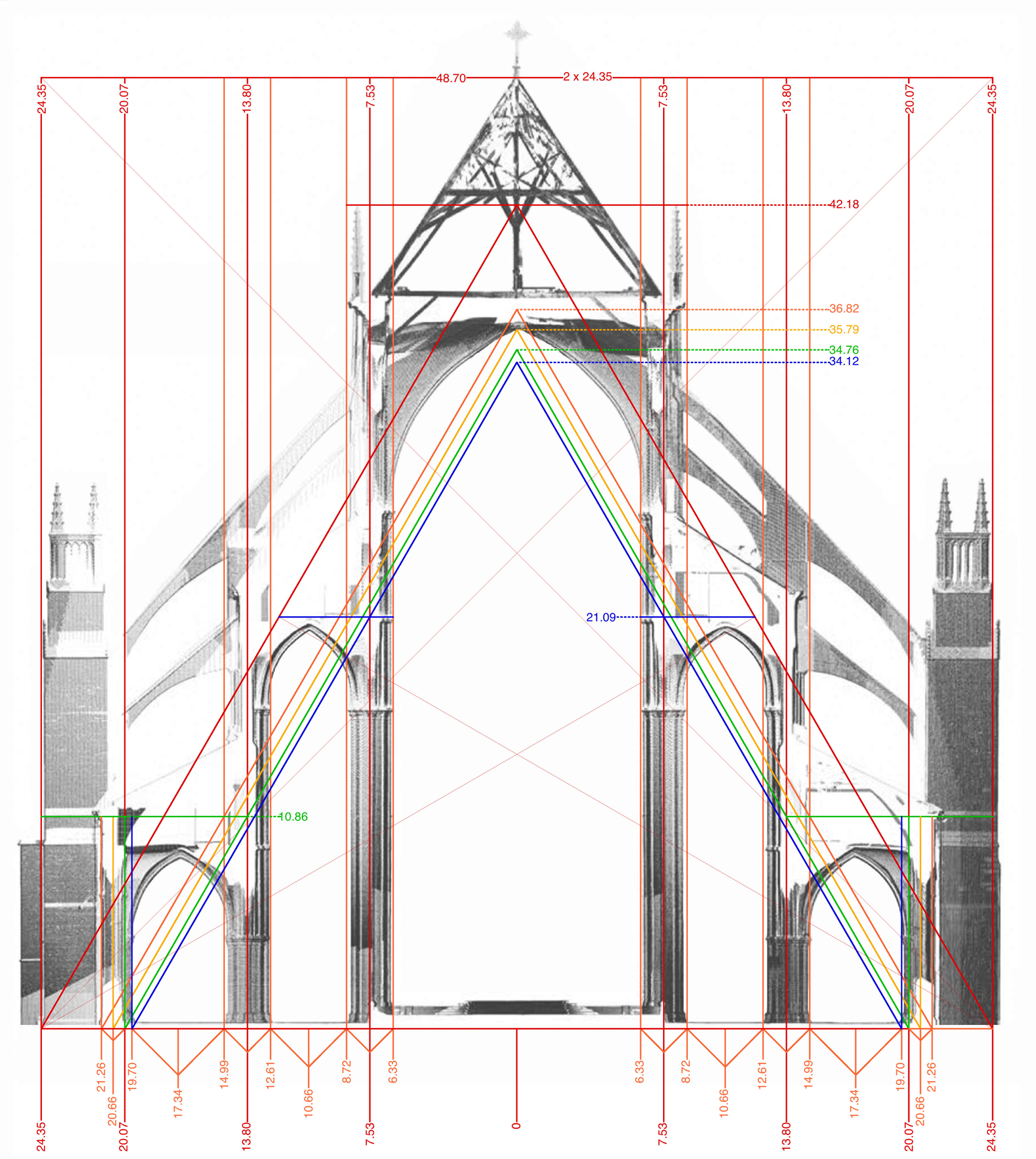
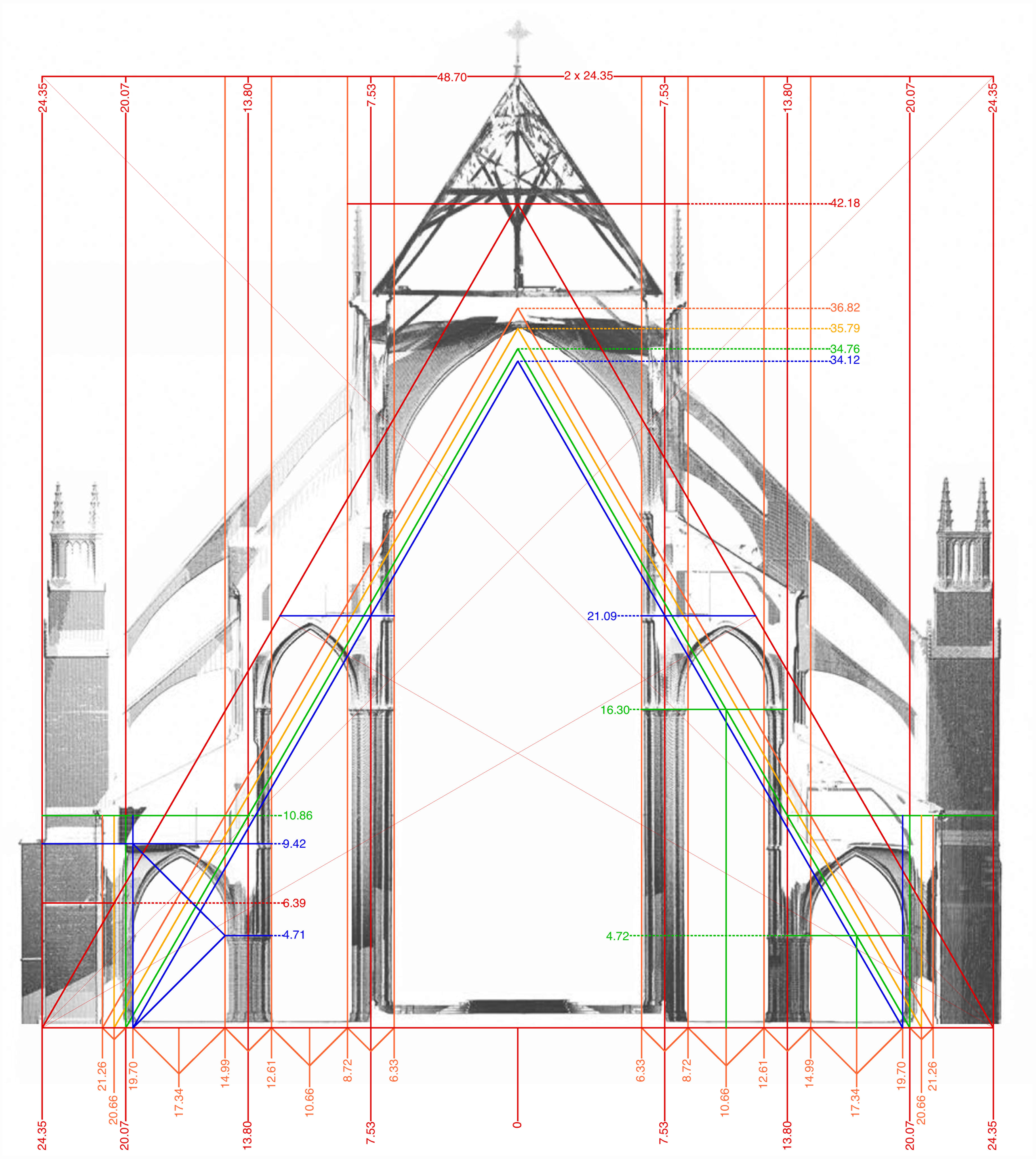
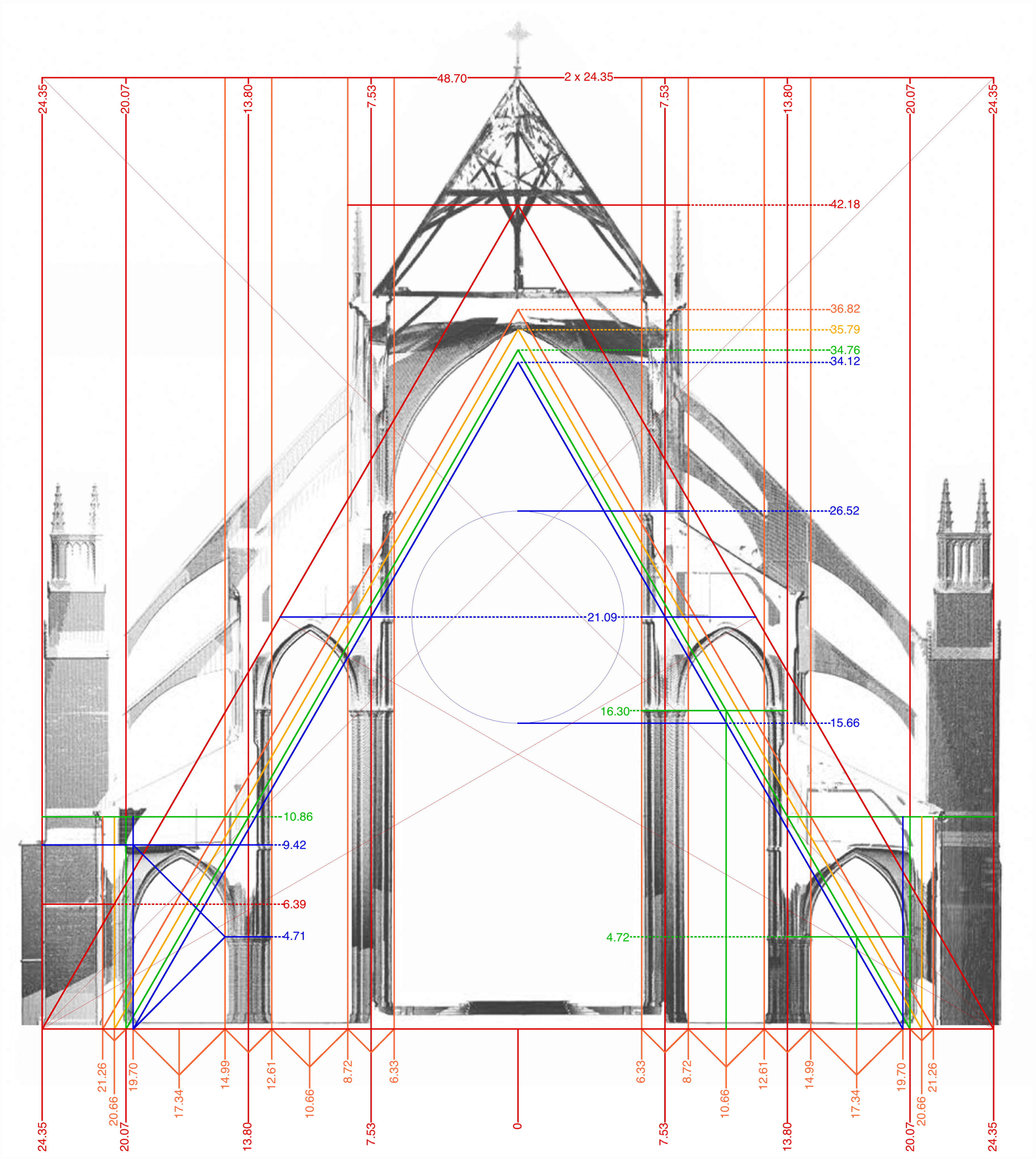
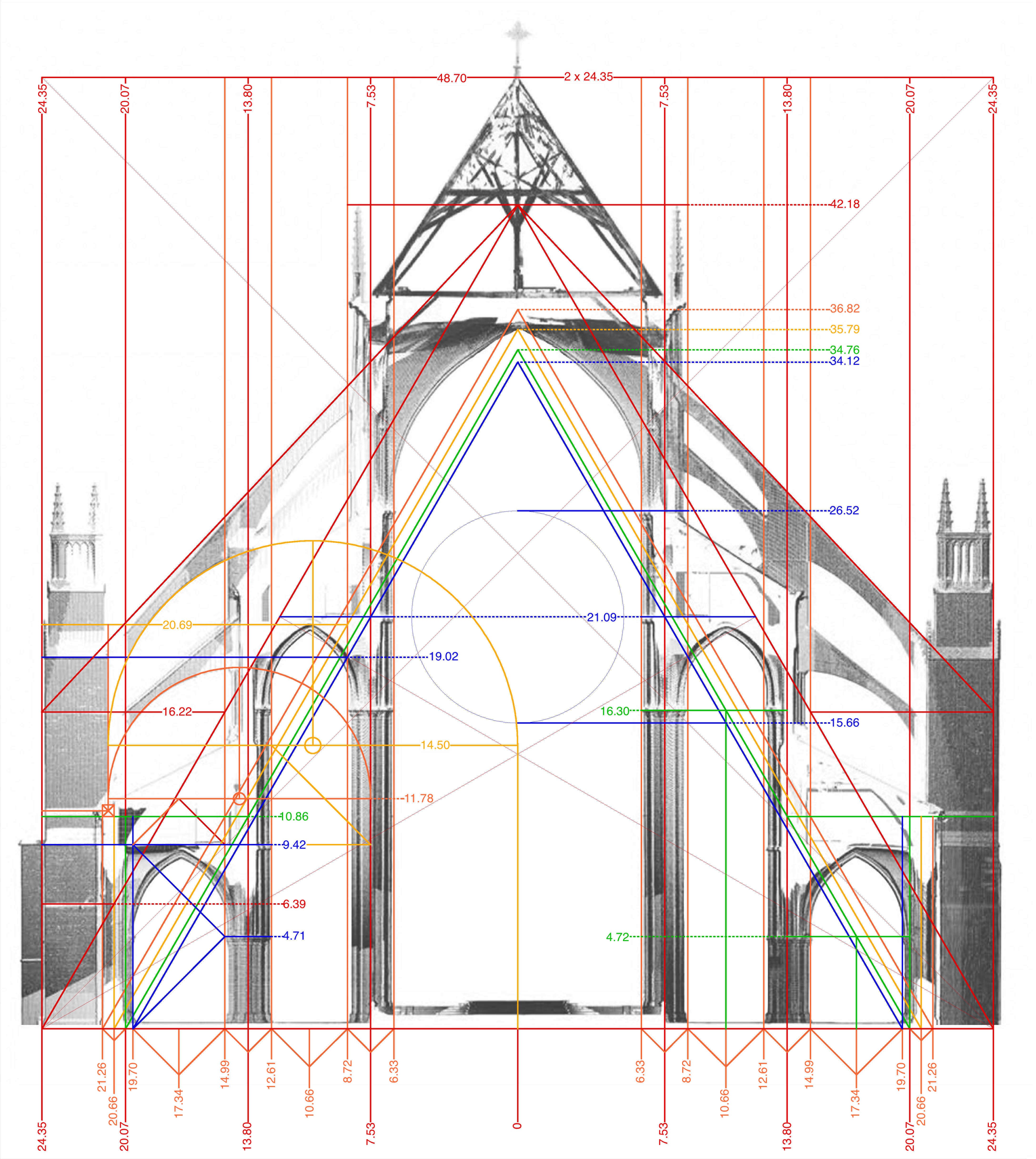
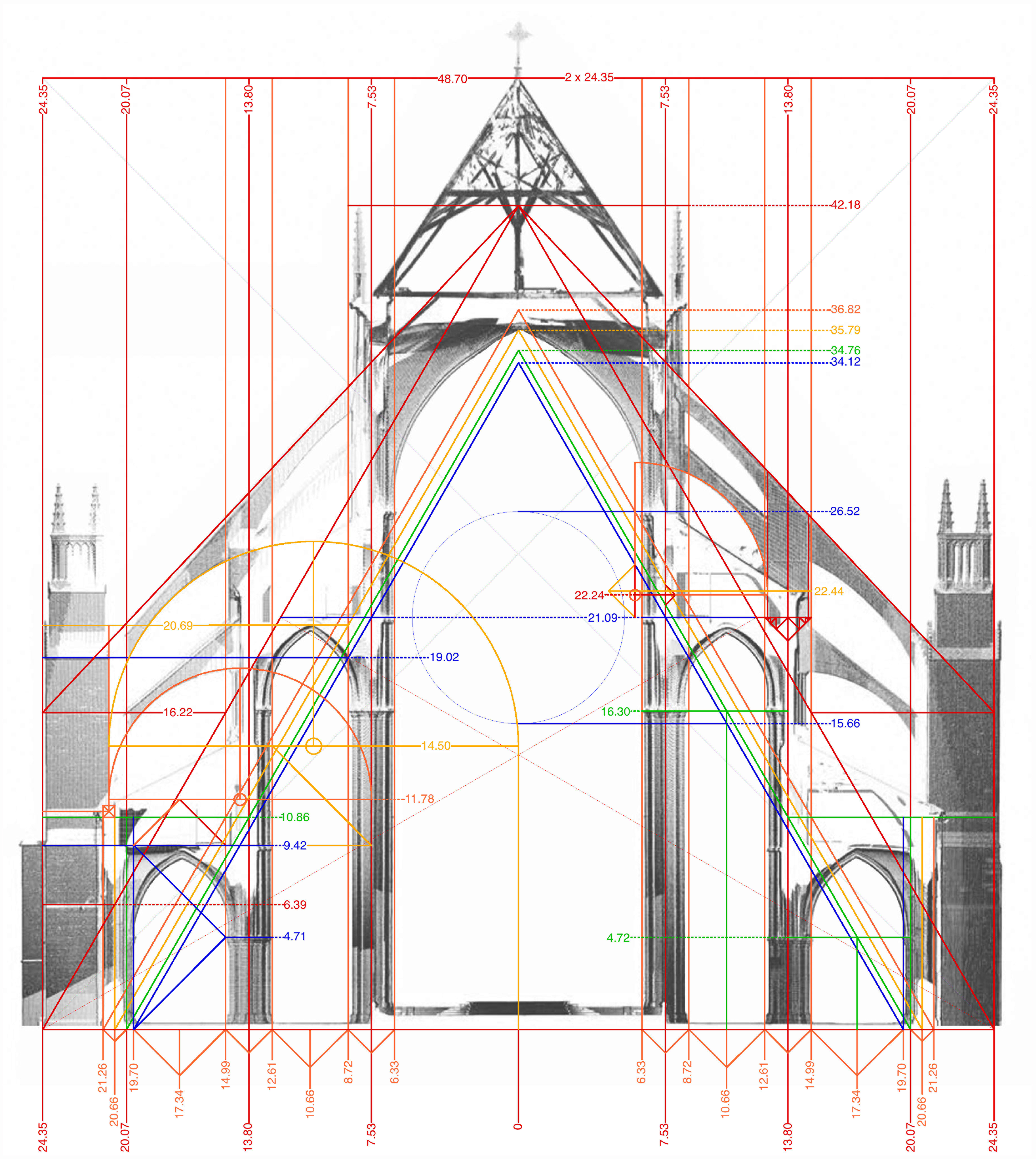
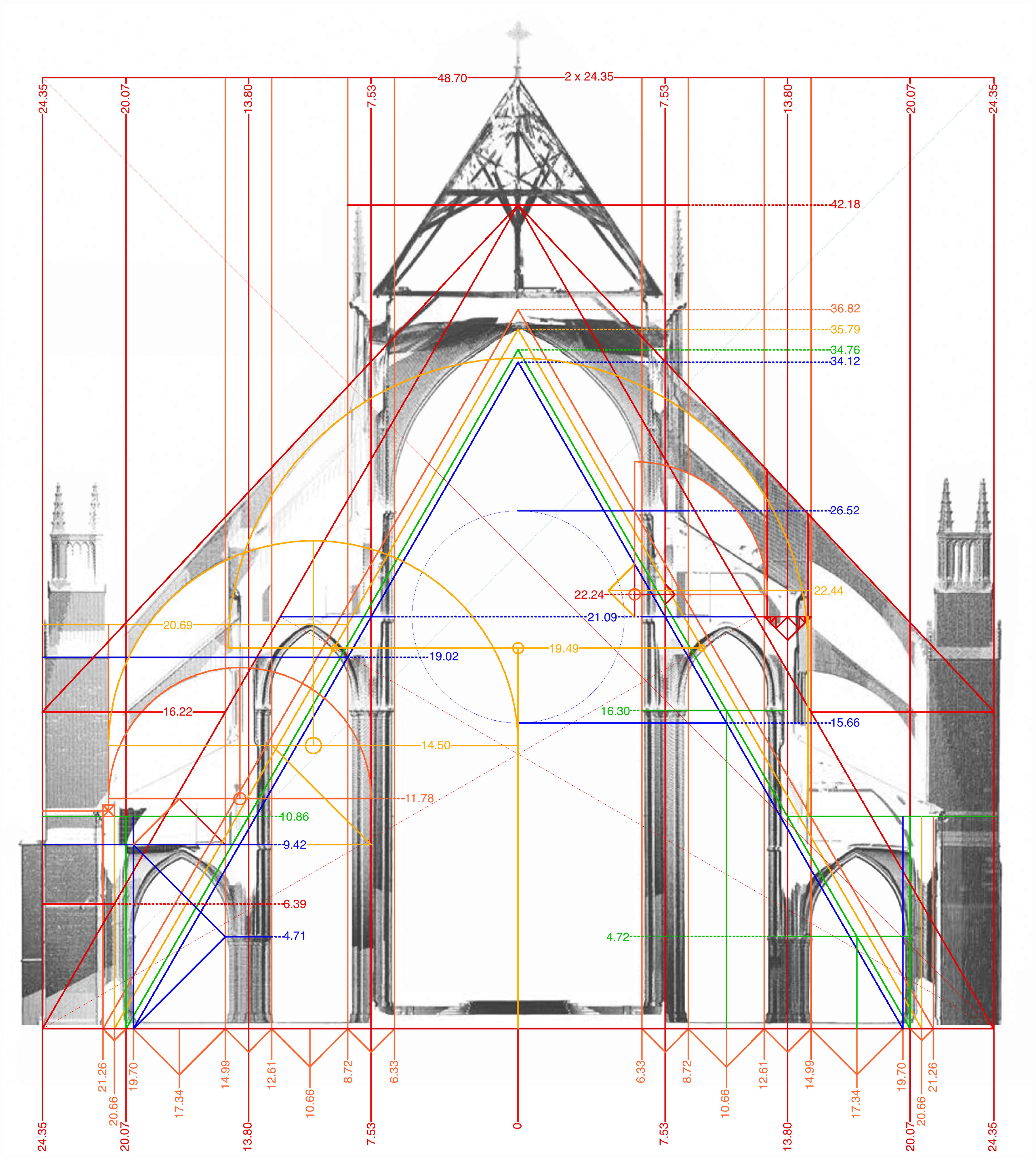
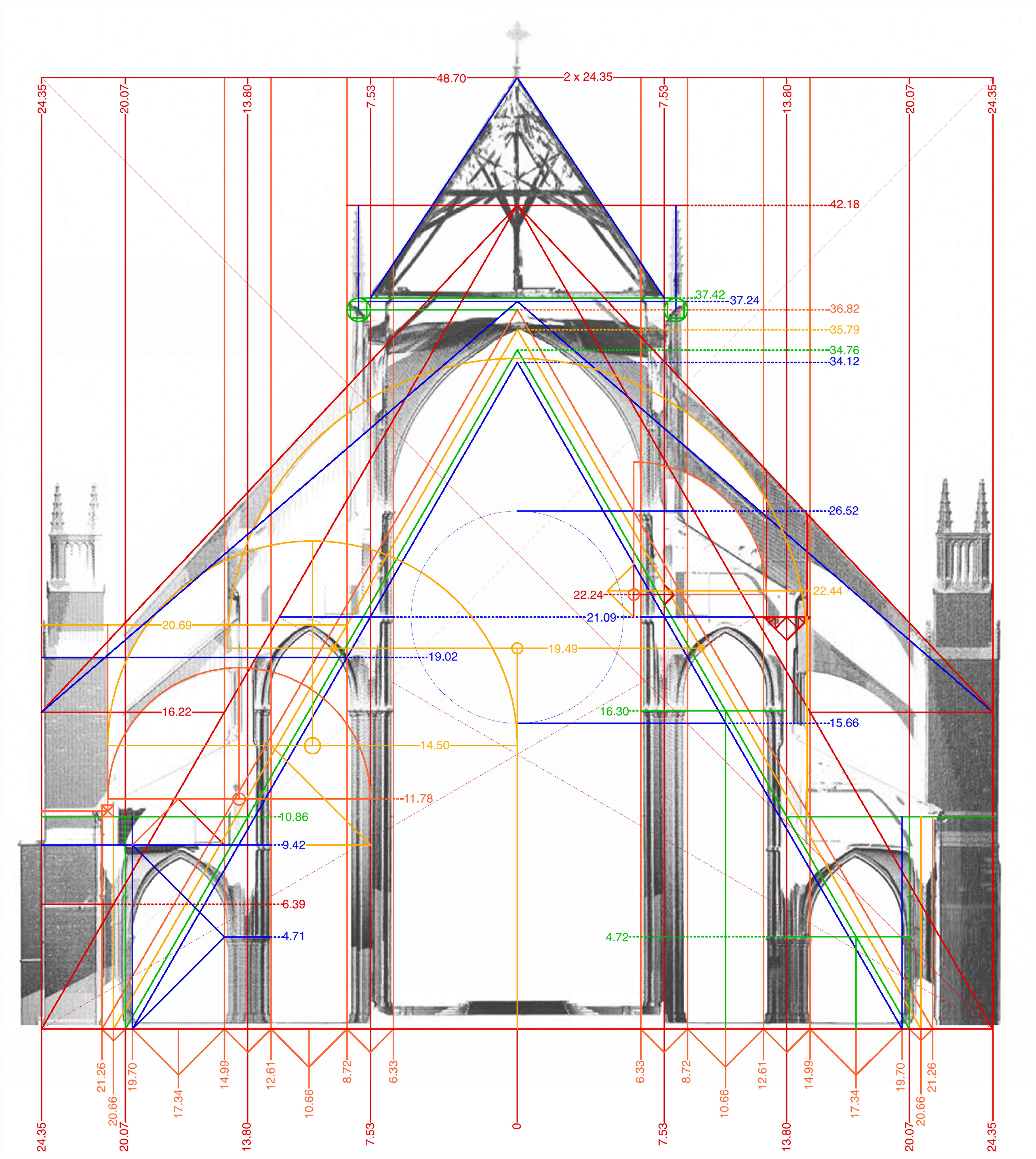
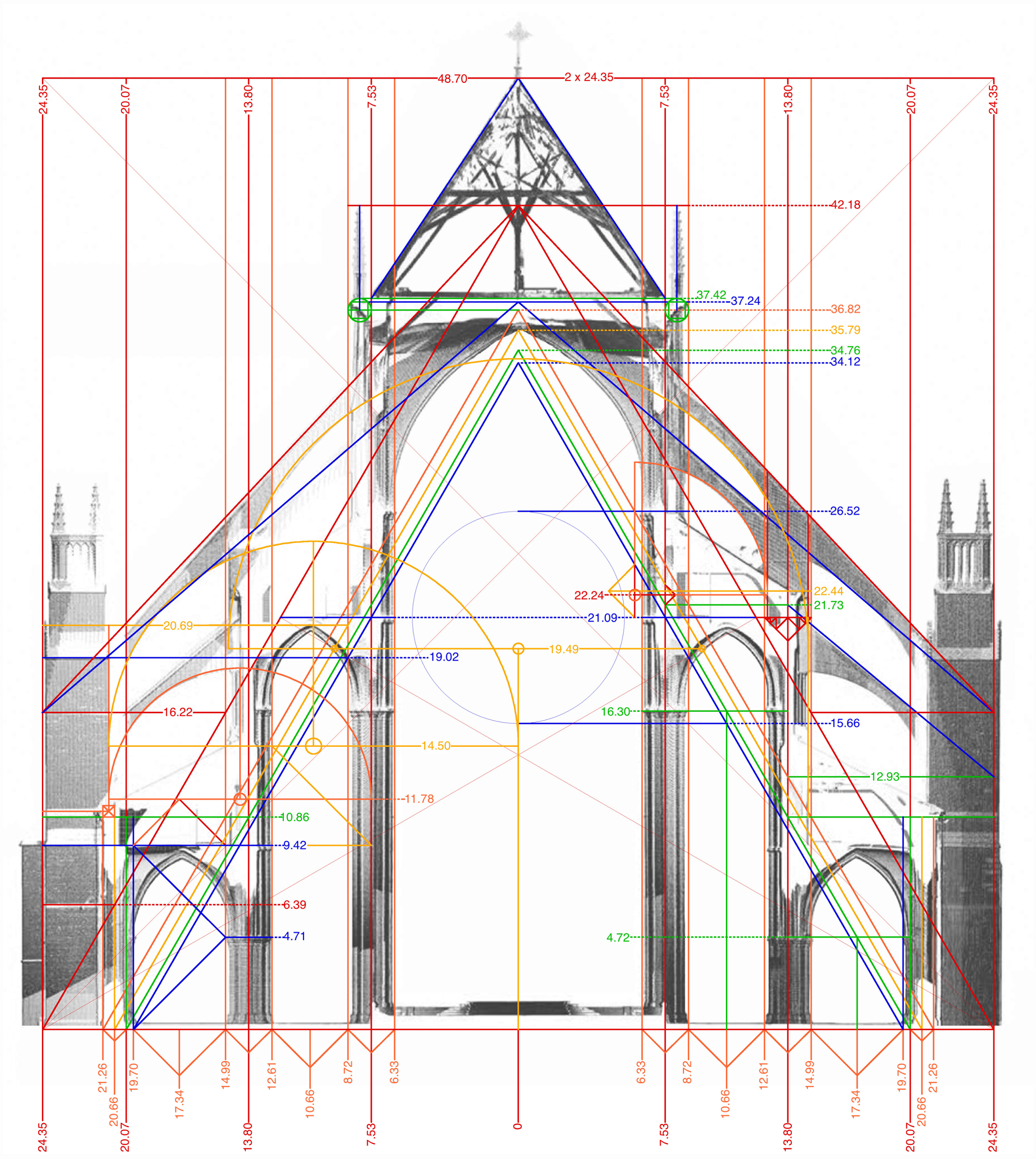
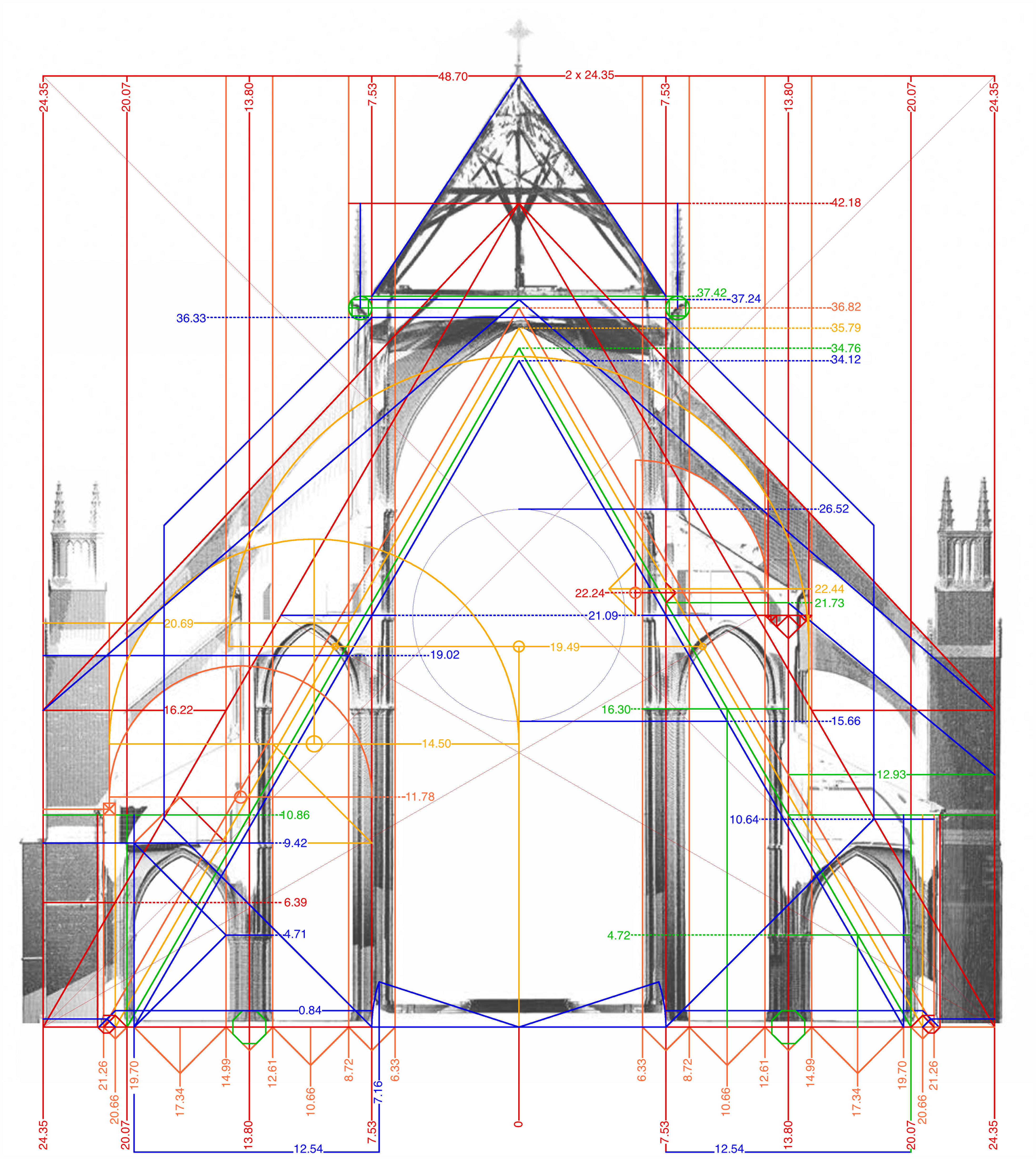
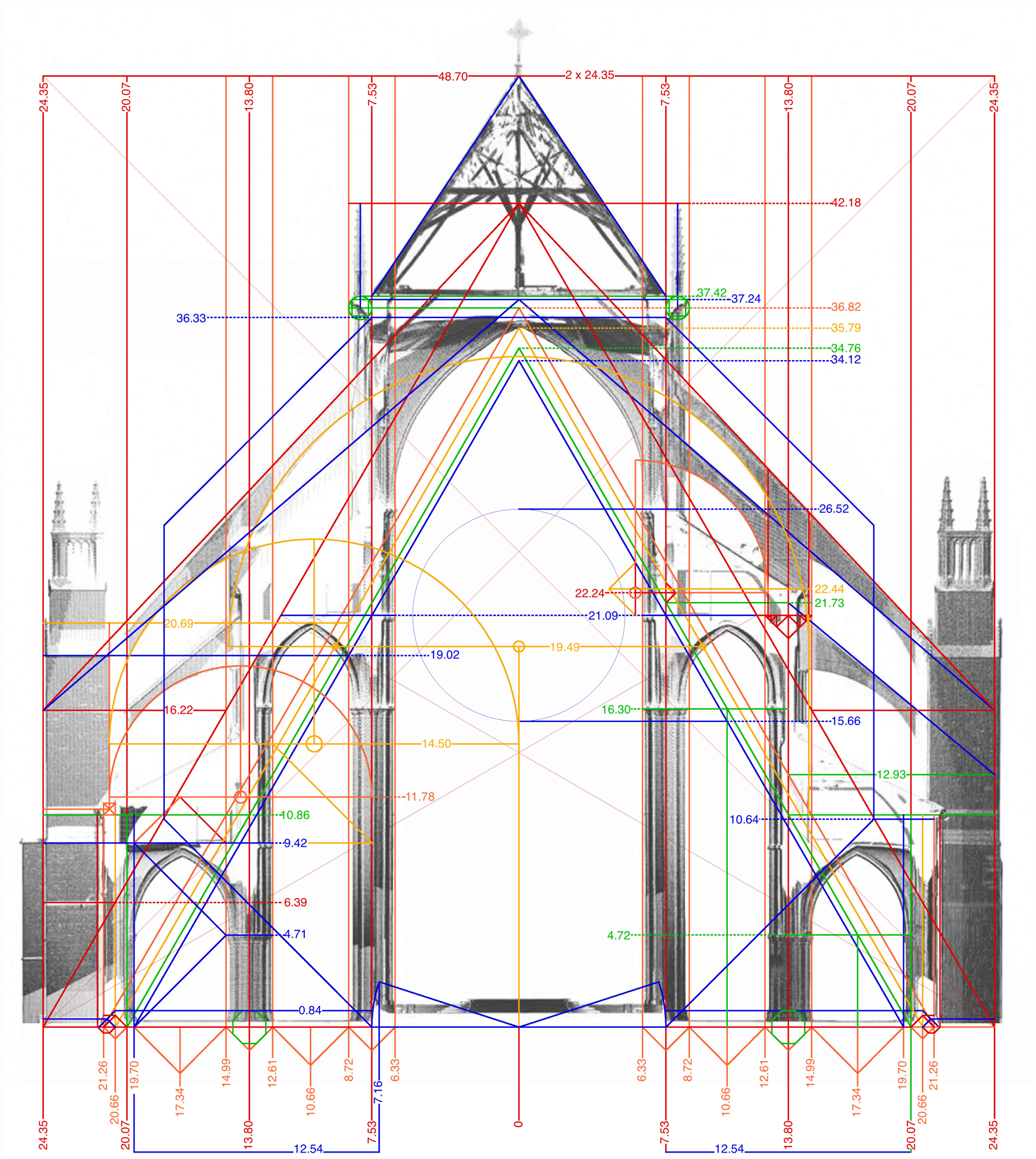
The decisions of the Bourges designer, and his likely use of the octagon alongside the square and the equilateral triangle, demand to be understood in their broader artistic context. The Bourges designer, for example, clearly drew inspiration from Notre-Dame in Paris, another cathedral planned with five aisles and an ‘ad triangulum scheme.’ It is surely significant, therefore, that the gutteral wall of Notre-Dame steps outward at a level corresponding to the height of a great octagon established on the base of the choir vessel, as the upper left quadrant of this graphic indicates. The whole section geometry of Reims Cathedral depends upon such a great octagon, as seen in the lower left quadrant of the graphic; the dotted line shows the originally planned curvature of the vaults, whose apex would have coincided with the top facet of the octagon, just as the top of the aisles coincide with its equator. The Reims designer made no use of the ‘ad triangulum’ scheme, and, where the Bourges designer set the height of his timber roof using a large framing square, the Reims designer unfolded the half-diagonal of the square framing his master octagon, thus creating a Golden Section relationship to set the height his roof. A few decades later, around 1231, the designer of the new nave at Saint-Denis returned to the more synthetic approach of the Bourges master, creating a nave vessel that fit neatly within a double square, while using equilateral triangles to set the proportions of the roof and the side aisles, and octagon-based schemes to set the baseline of the triforium, the baseline of the clerestory windows, and the height of the upper capitals. Each of these buildings deserves the kind of detailed geometrical analysis that has been given for Bourges in the preceding discussion. As these preliminary graphics already indicate, though, careful geometrical analysis can provide valuable information not only about the design of individual monuments, but also about their places in the larger history of Gothic architecture.